Delta (Dirac) function integral
I have a question about the integral of the $\delta$ (Dirac) function. Is the following correct?
$\int^{c}_{0} d\delta(x) = -\delta(0) = -\infty$
where c>0. Can we have a specific number for the result?
Are you trying to work out the integral
$$\int_0^a \delta(x)dx \textrm{ ?}$$
In that case, the expression you are trying to evaluate doesn't have a precise definition.
For example, the defining properties of the delta function are that:
- $\delta(x) = 0$ if $x\neq 0$.
- $\int_{-\infty}^{\infty}\delta(x) = 1$
These tell us that if $(a,b)$ is an interval containing $0$ (i.e., $a<0$ and $b>0$) then $\int_{-\infty}^a\delta(x)dx=0$ and $\int_b^{\infty}\delta(x)dx=0$, so $\int_a^b\delta(x)dx=1$.
On the other hand, if $[a,b]$ doesn't contain $0$, then $\delta(x)$ is the zero function over $[a,b]$ so certainly $\int_a^b\delta(x)dx=0$.
So if you're integrating from $0$ to $a$, does that contain the point $0$ or not? If you consider yourself integrating over $[0,a]$, then $0$ is contained in the interval, and if you think you're integrating over $(0,a)$, then $0$ is not contained in the interval.
One semi-satisfactory rigorous way of dealing with the delta function (since there is no function that actually satisfies (1) and (2) above) is to create a sequence of functions $\delta_n$ like these ones:
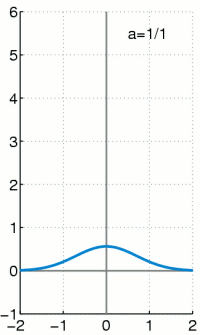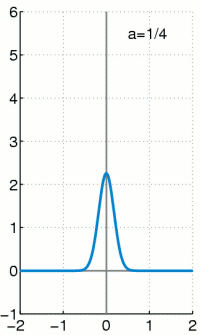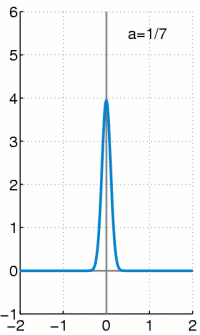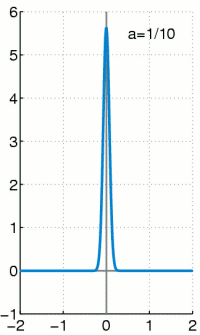
The sequence $\delta_n(x)$ converges to $0$ for all $x\neq 0$, and the integral from $-\infty$ to $\infty$ of each $\delta_n$ is $1$. We then define $\int_a^b f(x)\delta(x)dx$ to be the limit of the expression $\int_a^b f(x)\delta_n(x)dx$ as $n\to\infty$, which can be shown to be $f(0)$ if $(a,b)$ contains $0$ and $0$ if $[a,b]$ doesn't contain $0$.
If you try to work out $\int_0^a\delta(x)dx$ then you get that
$$\int_0^a\delta_n(x)dx=\frac{1}{2}\int_{-a}^a\delta_n(x)dx\to\frac{1}{2}\textrm{ as }n\to\infty$$
using the fact that the functions $\delta_n$ are continuous. However, this is an unsatisfactory answer for a number of reasons:
- The symmetry of the $\delta_n$ is not an essential part of their definition. I could quite easily choose $\delta_n$ that weren't symmetric, and still gave a valid definition of the delta function.
- Integrating the $\delta$ function should give either $1$ or $0$. Getting a value of $\frac{1}{2}$ is like bouncing a ball and saying that half of the impulse happened just before the bounce, and half happened just after.
- You're not supposed to integrate the $\delta$ function when one of the end-points is $0$.
I'm afraid that that's really the main problem here. Since the $\delta$ function doesn't actually exist as a function (all functions $\mathbb{R}\to\mathbb{R}$ which are zero everywhere except at a point have Riemann integral equal to $0$, and never $1$), there's no definition of what $\int_0^a\delta(x)dx$ means.
The $\delta$ function was originally designed for solving physics problems. Your problem is like the following: if you bounce a ball off a surface, the impulse from the surface can be modelled as being $0$ when $t\neq 0$ and having an integral over all time of $1$ (if $1$ is the impulse the table exerts on the ball). Your question is like asking what the total impulse given to the ball is starting at the point the ball hit the surface and finishing at some other time. There's no way to answer the question: if you start when the ball hits the surface, do you include the impuls from the surface or not? It's an impossible (and highly uninteresting) problem in physics.
Interestingly, one other 'definition' of the $\delta$ function is to let it be the Fourier series
$$\delta(x) = \frac{1}{2\pi}\sum_{n=-\infty}^\infty e^{inx}$$
Substituting this into the expression
$$\int_a^b f(x)\delta(x)dx$$
and integrating term-by-term gives you exactly the behaviour of the $\delta$ function. Then, if you try doing the integral
$$\int_0^a \delta(x)dx\leadsto\int_0^a \frac{1}{2\pi}\sum_{n=-\infty}^\infty e^{inx} dx$$
and integrate term by term, you do indeed get $\frac{1}{2}$. So maybe $\frac{1}{2}$ is as good an answer as you're going to get.